
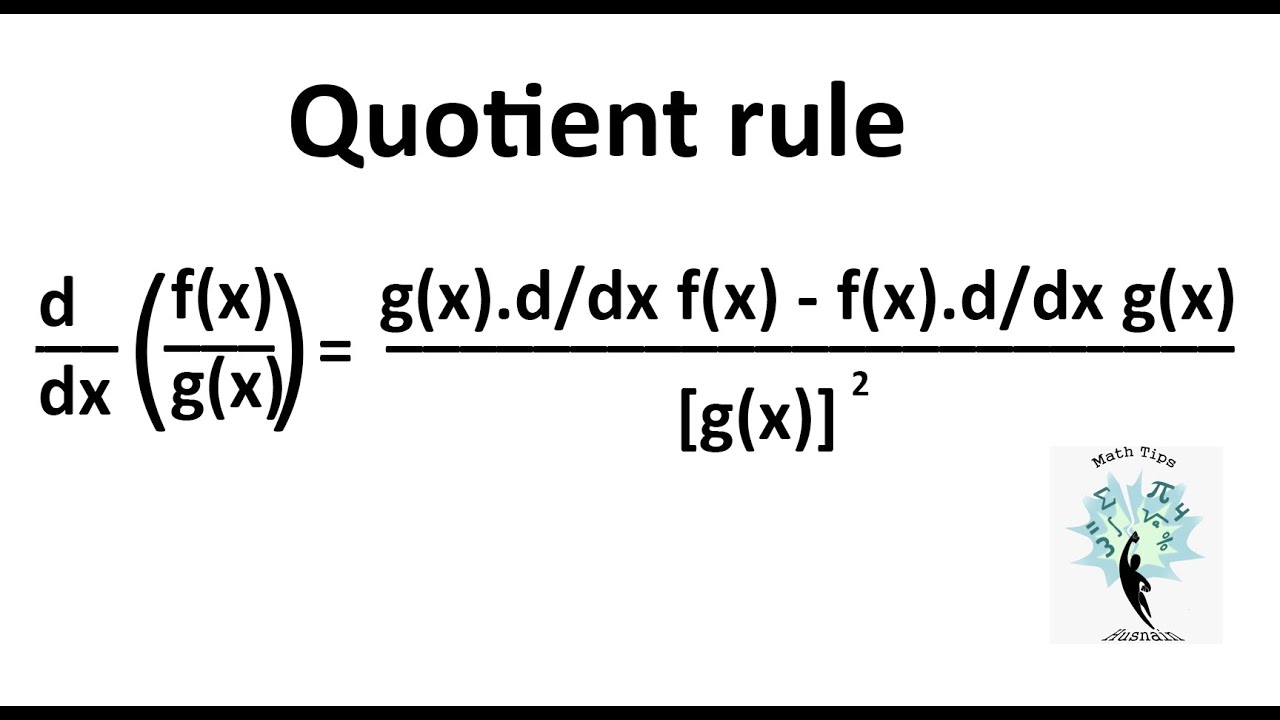
Extend the power rule to functions with negative exponents. Use the quotient rule for finding the derivative of a quotient of functions. We derive each rule and demonstrate it with an example. The quotient rule can be used to differentiate the tangent function tan (x), because of a basic identity, taken from trigonometry: tan (x) sin (x) / cos (x). Use the product rule for finding the derivative of a product of functions. Step 1: Name the top term f (x) and the bottom term g (x). Leibniz also proposed many important things in fields like probability theory, biology, geology, psychology and computer science.D d x = d d x ⋅ g ( x ) − f ( x ) ⋅ d d x 2 \dfrac 2 f ′ ( 4 ) g ( 4 ) − f ( 4 ) g ′ ( 4 ) start fraction, f, prime, left parenthesis, 4, right parenthesis, g, left parenthesis, 4, right parenthesis, minus, f, left parenthesis, 4, right parenthesis, g, prime, left parenthesis, 4, right parenthesis, divided by, open bracket, g, left parenthesis, 4, right parenthesis, close bracket, squared, end fraction. Sharing is caringTweetIn this post, we are going to explain the product rule, the chain rule, and the quotient rule for calculating derivatives. The quotient rule can be used to differentiate the tangent function tan (x), because of a basic identity, taken from trigonometry: tan (x) sin (x) / cos (x). Leibniz is credited alongside Newton for the invention of calculus. Basically, you take the derivative of f f ff multiplied by g g gg, subtract f f ff multiplied by the derivative of g g gg, and divide all that by g ( x ). He also proposed many theories of calculus like the fundamental theorem of calculus, Leibniz integral rule and Leibniz’s law. It is one of the basic, simple and widely used rule to differentiate equations. Example 2.14 Evaluating a Limit Using Limit Laws Use the limit laws to evaluate lim x 3(4x + 2). We now practice applying these limit laws to evaluate a limit. The notations for integration \(\int\) and differentiation \(d\) were defined by him. The quotient rule is a fundamental rule in differential calculus. Root law for limits: lim x a nf(x) nlim x af(x) nL for all L if n is odd and for L 0 if n is even and f(x) 0. Example 1 Differentiate each of the following functions. Let’s do a couple of examples of the product rule. Engraving of Gottfried Wilhelm Leibniz ( Source) The proof of the Quotient Rule is shown in the Proof of Various Derivative Formulas section of the Extras chapter.


 0 kommentar(er)
0 kommentar(er)
